Spectra and Spectral Lines

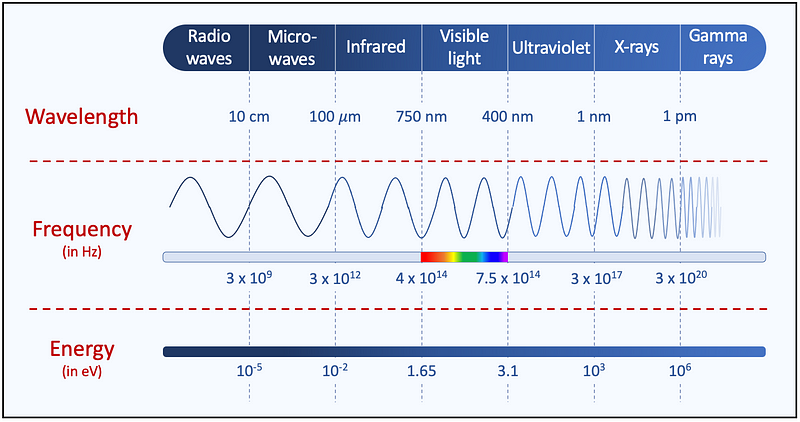
Light consists of oscillating electromagnetic fields that carry energy and can traverse empty space, which is why we can feel the Sun's warmth. Essentially, light is composed of energy waves, with their frequency—meaning the number of oscillations per second—spanning a continuous spectrum from very low (radio waves) to very high (gamma rays).

The wavelength of an electromagnetic wave is defined as the distance between two peaks or troughs and is inversely related to frequency: a lower frequency corresponds to a longer wavelength, while a higher frequency leads to a shorter wavelength. Additionally, shorter wavelengths (or higher frequencies) indicate that the light carries more energy.

The electromagnetic spectrum is divided into seven categories of radiation based on frequency (from lowest to highest): radio waves, microwaves, infrared radiation, visible light, ultraviolet radiation, X-rays, and gamma rays.

Stellar spectroscopy involves studying the light emitted by celestial bodies, where the light is dispersed into a spectrum of individual wavelengths using a diffraction grating. The resulting spectra can be categorized into three types: continuous, emission, and absorption spectra.

A continuous spectrum is generated by a gas, liquid, or solid (commonly referred to as a blackbody radiator) that exhibits no gaps in its spectrum. In contrast, an emission spectrum arises from a hot gas that emits light, characterized by a few colored lines on a dark background. An absorption spectrum is formed when light passes through a cooler gas cloud before being observed, resulting in a continuous spectrum with black lines superimposed. The lines in both emission and absorption spectra are termed spectral lines.

It is essential to remember that the gaps in an absorption spectrum occur at the same wavelengths where colored spectral lines are found in an emission spectrum. Instead of displaying the emission and absorption spectra as electromagnetic bands with spectral lines, they can also be represented graphically, plotting flux against wavelength, where flux signifies the luminosity or intrinsic brightness of a star, reflecting the energy received per unit of time across a specified area.

Shining Light on Matter

The spectral lines illustrated arise from the interaction of matter with light. Specifically, the atoms constituting matter can absorb and re-emit electromagnetic waves, but this occurs only when the energy of incoming light matches the energy difference between two specific levels in the atom.

In essence, an atom features a positively charged nucleus around which negatively charged electrons orbit at defined energy levels known as orbits, maintaining a relatively fixed distance from the nucleus. Electrons may transition to lower energy orbits closer to the nucleus or ascend to higher energy bands further away. Each orbit, or electron shell, comprises a specific number of energy levels called subshells.

When an electron transitions from a higher to a lower energy subshell, it releases energy equivalent to the energy difference between the two subshells, emitting this energy in the form of electromagnetic radiation, or light. Conversely, an electron moving to a higher energy subshell absorbs energy from incoming electromagnetic waves. These two processes are termed emission and absorption of light, respectively.

Atoms of different chemical elements, such as iron (Fe) or magnesium (Mg), differ in the number of protons in their nucleus, which affects the strength of the electrostatic force drawing protons and electrons closer together. Each element has a unique number of protons, and consequently, the energy levels associated with each subshell and the energy differences between them are distinct.

Thus, if the energy associated with a specific wavelength of electromagnetic radiation matches the energy difference between two subshells in an atom, that wavelength is inherently linked to that particular subshell transition. Each element produces a unique set of wavelengths based on all possible electron transitions within its atoms.

This characteristic pattern of spectral lines is crucial in stellar spectroscopy as it reveals which chemical elements are absorbing or emitting specific wavelengths of light.

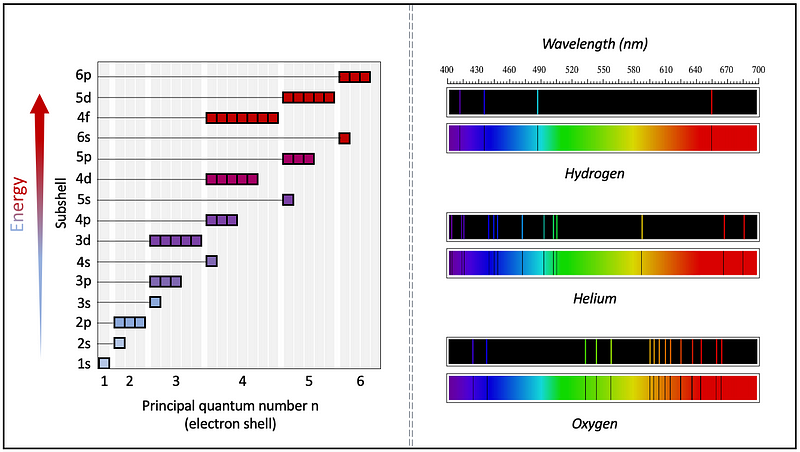
It is important to note that spectral lines in the infrared, visible light, and ultraviolet regions primarily arise from energy transitions of valence electrons—the outermost electrons—rather than the inner electrons close to the nucleus.

For instance, the valence electron in sodium (Na) and hydrogen (H) can undergo various energy transitions among different subshells, where the lowest energy state (ground state) for sodium corresponds to the subshells 3s and for hydrogen to 1s.

Moreover, as more protons reside in a nucleus, leading to a heavier element, there are also more electrons surrounding the atom. In a neutral atom, the number of protons matches that of electrons, resulting in a greater number of spectral lines due to increasing energy transitions between subshells. However, this complexity makes spectral interpretation progressively challenging, as the spacing between energy levels becomes narrower.

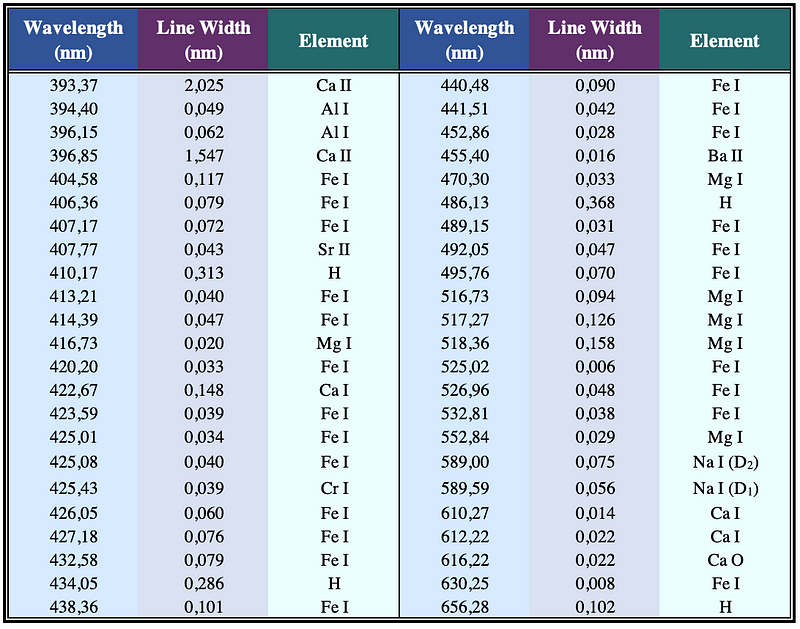
Table 1 further illustrates the unique relationship between wavelengths and specific chemical elements, providing a selection of wavelengths and corresponding spectral line widths for elements including calcium (Ca), aluminum (Al), iron (Fe), strontium (Sr), hydrogen (H), magnesium (Mg), chromium (Cr), barium (Ba), and sodium (Na). The Roman numeral I indicates a neutral atom, while II denotes an ionized atom.

Starlight Spectra

Light generated in a star's core, resulting from the fusion of hydrogen (H) and helium (He) atoms during stellar nucleosynthesis, travels toward the star's outer regions. The light does not follow a straight path; instead, it is scattered, becoming visible only after passing through the star's innermost atmospheric layer, the photosphere.

Nonetheless, the electromagnetic waves must traverse several outer gaseous layers before reaching empty space. Some wavelengths are absorbed by the atoms or ions in these layers, producing either an absorption or emission spectrum—ions are atoms that have lost or gained electrons, resulting in charged particles.

Stellar spectroscopy enables us to identify the chemical elements present in a star's atmosphere upon receiving its light.

For example, Figure 6 depicts the absorption spectrum of light from our Solar System's star, the Sun, indicating the chemical elements associated with several prominent spectral lines. Note that the molecular oxygen (O?) spectral lines at the red end of the spectrum result from sunlight absorption in the Earth's atmosphere, not the Sun's.

The width of a spectral line, or the strength of its profile in a flux versus wavelength graph, is influenced by the number of atoms involved in the corresponding energy transition. The more atoms or ions of a specific element that absorb a given wavelength, the broader the spectral line.

However, the number of atoms is not the sole factor affecting spectral line width. Other variables include the star's atmospheric temperature (discussed further in the section "Heat Waves Exposed"), pressure, quantum mechanical phenomena, the frequency of atomic collisions (gas density), and the star's rotation rate (refer to the subsection "Spinning Stars").

These various factors lead to diverse spectral appearances, as shown in Figure 7, which presents the absorption spectra of eleven stars in the visible light region.

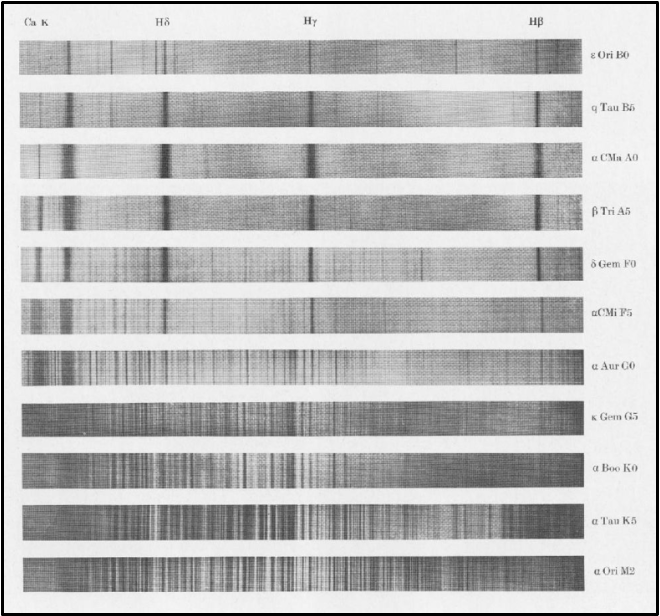
Despite the broad spectrum of stellar appearances, astronomer Cecilia Payne discovered that most stars share a similar atmospheric chemical composition: approximately 71.0% hydrogen (H), 27.1% helium (He), and 1.9% comprised of oxygen (O), carbon (C), and other heavier elements. In terms of atom counts, stars are primarily made up of 91.2% hydrogen (H), 8.7% helium (He), and 0.1% other elements including oxygen (O), carbon (C), nitrogen (N), silicon (Si), magnesium (Mg), neon (Ne), iron (Fe), and sulfur (S).

Payne's research established that the relative proportions of atoms at each energy level—ground state, first excited level, and so on—must be calculated for each element to accurately determine a star's chemical composition, rather than relying on line widths or the number of spectral lines.

Heat Waves Exposed

As previously mentioned, numerous factors influence a spectrum's profile. Particularly, the temperature of the photosphere significantly impacts the strength of absorption lines, revealing more about a star's surface temperature than its chemical composition.

Higher temperatures, defined as the average kinetic energy of a particle swarm, result in thermally excited electrons in atoms ascending to higher energy levels. Consequently, the relative fraction of spectral lines related to lower energy subshells diminishes—intensity is weakened.

Thus, the absence or reduced intensity of a specific spectral line does not imply that the corresponding chemical element is absent or less abundant in the star's atmosphere. Conversely, a greater number of spectral lines or a stronger profile does not necessarily indicate higher abundance.

Figure 8 illustrates how varying photosphere temperatures affect line profile strength. For the hottest stars (top two lines), most hydrogen (H) and metal atoms, such as magnesium (Mg), sodium (Na), and calcium (Ca), become ionized, lacking electrons for energy transitions and thus resulting in a weak spectral profile.

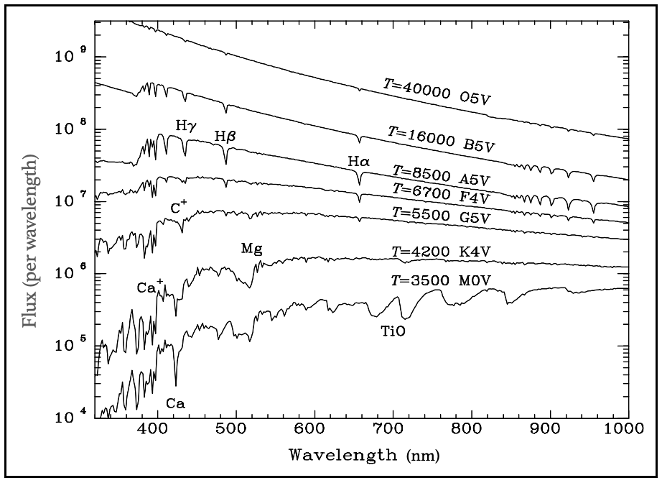
For hydrogen (H), this means relatively few atoms absorb lower energy wavelengths, since temperature is an average measure; some atoms will have lower temperatures.

As illustrated in Figure 5, the only lower energy level in hydrogen (H) that absorbs light in the optical region is the level n=2, corresponding to wavelengths of 656 nm (n=3), 486 nm (n=4), and 434 nm (n=5). Figure 8 indicates that these optical wavelengths result in only faint hydrogen (H) profiles for the hottest stars.

In stars with medium temperatures (third to fifth lines from the top in Figure 8), the surface temperature is optimal for most hydrogen (H) atoms to reside in the second energy level (n=2), resulting in a stronger spectral profile in the optical region, particularly for the line at T=8,500K.

For cooler stars (the two bottom lines), the hydrogen (H) line profile weakens again, as the lower temperature causes atoms to occupy mostly their ground state (n=1), absorbing ultraviolet wavelengths (around 100 nm) rather than optical wavelengths.

In contrast, the spectral profile of metal atoms strengthens in cooler stars since their ground state energy level absorbs optical wavelengths. Additionally, the presence of molecules, such as titanium oxide (TiO) in the T=3,500K line, contributes to a more pronounced spectral profile due to its absorption in the optical-infrared border region (700–900 nm).

Labelling Stars

Stars are categorized into seven classes based on their surface temperatures (from lowest to highest): M, K, G, F, A, B, and O. Each class letter is followed by two indices: a number from 0 to 9 (with 0 indicating the highest temperature) and a Roman numeral from I to VII (with I denoting the strongest luminosity).

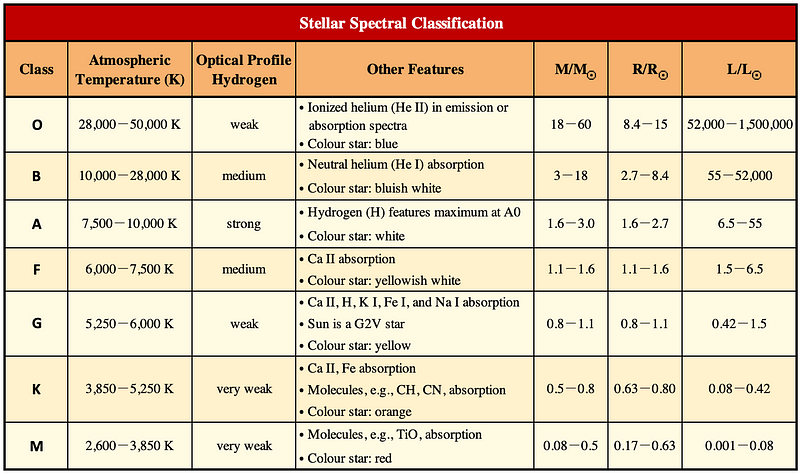
For instance, the Sun is classified as a G2V star with a surface temperature of approximately 5,800K, Alnitak Aa in the Orion constellation is an O9.5Iab star at 30,000K, Aldebaran in Taurus is a K5III star at 4,000K, and Sirius in Canis Major is an A1Va star at 9,940K. Note that the luminosity class is often further specified by three sublevels: a, ab, and b (in descending order).

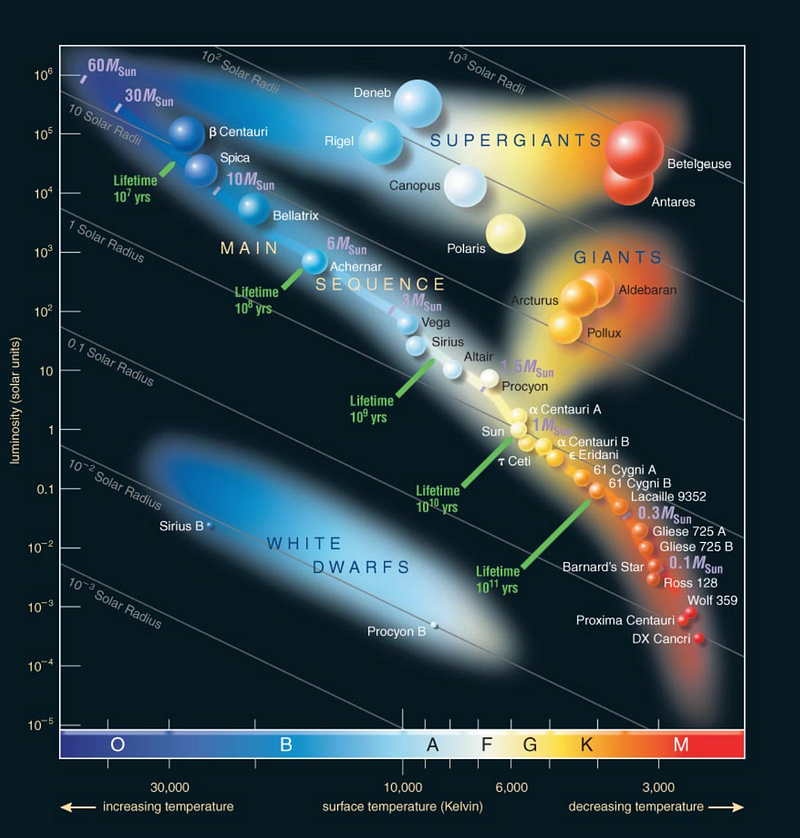
Generally, stars within the same class exhibit similar physical properties, such as mass, radius, and luminosity. Moreover, trends exist among these physical parameters; for example, brighter stars tend to be larger within a given class, and more massive stars are typically hotter and more luminous. When considering stellar age, these relationships can be represented in a graph known as the Hertzsprung-Russell Diagram, demonstrating that some stars may be very hot and small yet faint (e.g., Sirius B), while others are cold, large, and bright (e.g., Betelgeuse).

Line Widths and Profile Shapes

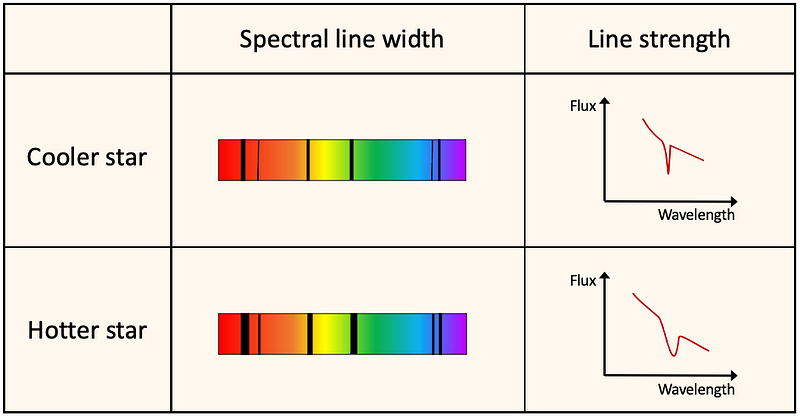
In addition to the relative effect of surface temperature on line strength (as discussed earlier), temperature also has an absolute impact. Spectral lines in hotter stars are broader compared to those in cooler stars—alternatively, the line profiles in flux versus wavelength graphs are less sharp and more spread out.

This phenomenon is attributed to thermal Doppler broadening, which arises from the relative motion of atoms or ions absorbing incoming light. The Doppler effect indicates that waves emitted by an object moving toward an observer appear compressed (shorter wavelengths) while those from a receding object are perceived as stretched (longer wavelengths), resulting in a shift toward the blue or red end of the spectrum, respectively.

As atoms or ions move within a gas, they all contribute to a Doppler shift to some degree. However, those in a hotter gas move more rapidly, causing a more pronounced Doppler effect. Thus, the wavelengths of atoms or ions moving away from us appear red-shifted, while those moving toward us appear blue-shifted.

The overall result of the increased motion of atoms or ions in a star's atmosphere—due to higher surface temperatures—is broader spectral lines.

Beyond line width and strength, surface temperature also alters the entire shape of a spectrum. A higher (or lower) temperature shifts the peak of a line's profile in a flux versus wavelength diagram toward shorter (or longer) wavelengths.

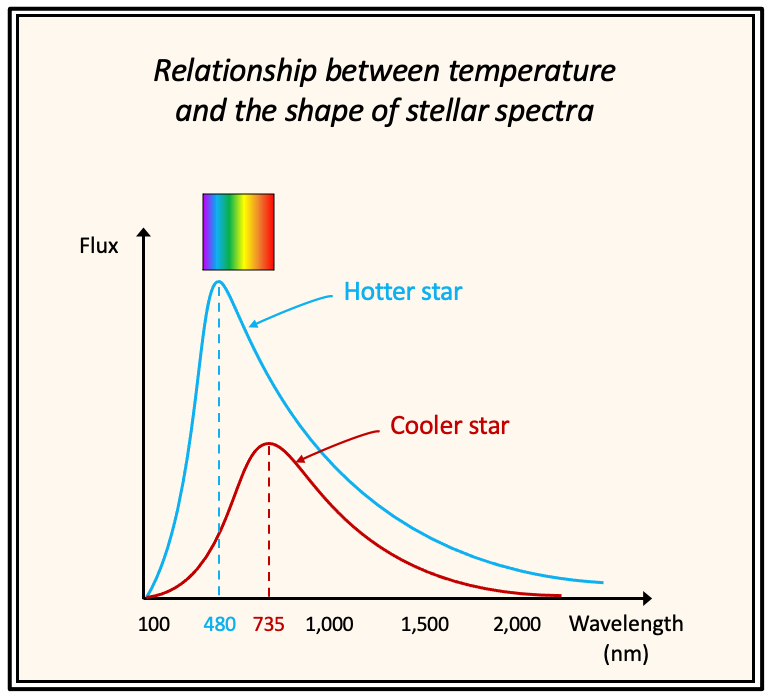
This occurs because rising temperature results in increased energy radiation, elevating the peak flux (and luminosity) while shifting that peak toward the blue end of the optical spectrum, as electromagnetic waves with shorter wavelengths carry more energy. In a flux versus wavelength graph, the total energy radiated corresponds to the area under the curve.

Regarding stellar spectral classification (see earlier subsection "Labelling Stars"), this relationship elucidates why hotter B stars appear bluish-white, whereas cooler M stars appear red.

Unscrambling Stellar Motion

In the previous subsection "Line Widths and Profile Shapes," the Doppler effect illustrated how a star's surface temperature influences spectral line characteristics. However, this motion-related effect also provides insights into various physical quantities and astrophysical phenomena. For instance, the Doppler effect accounts for the direction in which stars, or entire galaxies, are moving.

When a star moves toward us, its light exhibits a shift in spectral lines toward shorter wavelengths—electromagnetic waves appear compressed. Conversely, a star moving away from us results in red-shifted spectral lines, where wavelengths appear longer.

The difference between observed wavelengths and those of a stationary reference star, like the Sun, allows us to calculate the star's velocity along the observer's line of sight—this is termed radial velocity. A greater wavelength gap indicates a higher velocity of the moving star.

In addition to radial velocity, stars also possess transverse velocity, or proper motion, which is perpendicular to the observer's line of sight. However, proper motion does not affect spectral line positioning and is not further elaborated upon here.

Figure 12 illustrates how the relative motion of a star affects the position of its hydrogen (H) spectral lines within its absorption spectrum. Since most stars consist of approximately 71.0% hydrogen (H), they typically feature hydrogen lines in their optical spectra, provided the surface temperature is adequate.

Aside from Doppler redshift from a star's receding motion, another phenomenon—cosmological redshift—occurs with distant stars or galaxies, linked to the expansion of the Universe. The farther away a star or galaxy is, the more pronounced the wavelength shift becomes due to greater expansion of spacetime, leading to a larger redshift. Additionally, greater distance implies higher receding velocity, which relates to Hubble's Law.

Spinning Stars

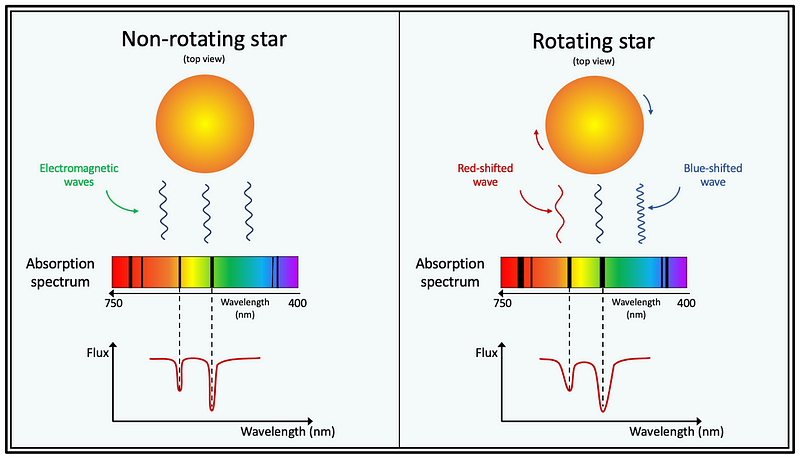
The Doppler effect also sheds light on rotating stars. In such stars, one side (e.g., the left side) moves toward us while the opposite side (right side) recedes. If we consider the rotation axis perpendicular to our line of sight, the light from the left side appears blue-shifted, while that from the right side appears red-shifted.

The overall outcome is broadened spectral lines, a phenomenon known as rotational broadening. The faster a star rotates, the thicker its spectral lines appear. By analyzing the wavelength shifts, we can calculate the star's rotation rate—i.e., the time required for a complete rotation.

Generally, hotter stars rotate more rapidly than cooler ones. For example, the Sun (a G2V star) has an average rotation period of 27 days, Alpha Caeli A (an F2V star) rotates in about 1.4 days, while Altair (an A7V star) completes a rotation in approximately 9.3 hours.

Treasures in a Flickering Disguise

This article examined how the properties of matter in a star's outer layer, atmospheric temperature, and both relative and rotational motion can be inferred from the light they emit.

However, this exploration barely scratches the surface, as stellar spectra can reveal even more information, including the presence of magnetic fields, identification of binary star systems, insights into the structure of galaxies and dark matter, and the size of the Universe through Hubble's constant.

Unlike the information on your passport, the wealth of data embedded in starlight will forever remain outdated, as electromagnetic waves travel through a vacuum at a finite speed—the speed of light. Therefore, when we gaze at the stars, we are looking into the past, given the vast distances separating us.

Nonetheless, this limitation will not diminish our desire to decode the flickering messages that the Universe communicates to us.