generate a new title here, between 50 to 60 characters long
Written on
Physics Showdown: Tesla Cybertruck vs. Porsche 911
The intriguing claim that “The Cybertruck outperforms a Porsche 911 while towing another 911” certainly grabs attention. However, is this assertion valid? The answer might not be straightforward.
In a recent Tesla video, a competitive race between the Cybertruck and a 911 was showcased, where the Cybertruck was seen towing a 911.
Yet, as pointed out by Engineering Explained's Jason Fenske, this race was not a quarter-mile test but merely an eighth-mile run. This revelation raises the subsequent question: would the Cybertruck maintain its lead over a full quarter-mile? According to Jason, the answer is no. It’s surprising that Tesla misrepresented the distance of their video—it’s quite misleading.
Although I appreciate Jason's analysis, I found some aspects lacking. So, I’ve decided to conduct my own assessment for entertainment.
Let's begin with a video analysis of the race featured in Tesla's clip. Similar to Jason's method, I'll determine the eighth-mile completion times for both vehicles. You don't need to manually count frames as he does; software like Tracker Video Analysis can streamline this process. Using it, I found the Cybertruck’s time to be 8.541 seconds, while the Porsche clocked in at 8.708 seconds. We can further utilize these times to calculate average acceleration shortly.
Next, I will assess the speed of each vehicle upon crossing the eighth-mile mark. I can extract position-time data from the video, but I must first establish the scale. Using the known length of 5.68 meters for the Cybertruck, I generated the following position-time plot.
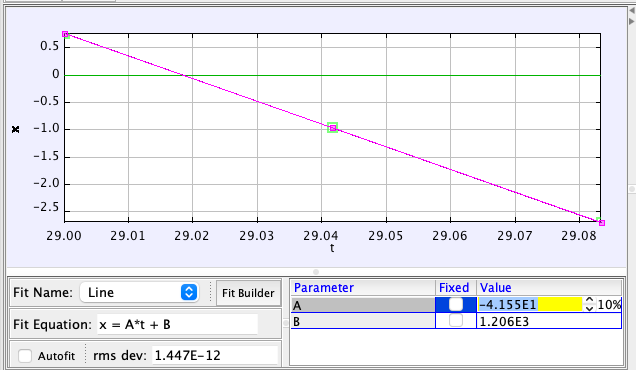
Although we only have three data points, they suffice. The x-velocity corresponds to the rate of change of position, and the slope of this line reveals a speed of 41.5 meters per second (approximately 92.8 mph). Now, considering the Porsche 911, which is closer to the camera, I need to rescale using its length of 4.52 meters. Here’s the resulting plot.

While it may appear that the Porsche is faster at 43.58 m/s (about 97.5 mph), this conclusion is tentative due to having only two data points. Moreover, the visibility of the 911 in the video isn’t ideal, which could mean its speed is slightly overestimated.
Constant Acceleration Analysis
What if we assume the eighth-mile times are accurate and both vehicles achieved constant acceleration during the race? Under such conditions, the average velocity during the run is simply the mean of the initial and final velocities. We can also express average velocity as the distance traveled (eighth of a mile) divided by the elapsed time.

Given that the initial velocity (v₁) is zero, the final velocity can be determined using the formula:
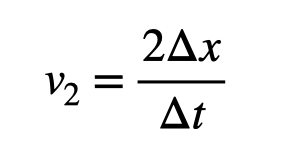
Plugging in the times and distance yields a final speed of 47.11 m/s (about 105.4 mph) for the Cybertruck and 46.20 m/s (around 103.4 mph) for the Porsche. This outcome appears plausible.
Constant Power Model Analysis
How can we project the Cybertruck's performance over a quarter-mile while towing a Porsche 911? Let’s compile our data: the Cybertruck's eighth-mile time is 6.94 seconds at 99 mph (according to Engineering Explained), while its towing time is 8.541 seconds at 92.8 mph. Its quarter-mile time without towing is 11.0 seconds at 118 mph, and the Porsche 911 T's quarter-mile time is 11.5 seconds at 120 mph.
To proceed, we need the Cybertruck's mass, which is 3104 kg, and an estimated mass for the Porsche 911 T (with the trailer) of 1600 kg. This information will allow us to model the motion of a vehicle across the quarter-mile track.
The simplest approach assumes that a drag racing vehicle operates under constant power. Since power represents the rate of energy change, this power contributes to increasing the vehicle's kinetic energy.

While this model is not flawless, it helps illustrate how added mass might influence race times. By dividing the vehicle's motion into short intervals, I can apply constant power to determine the velocity at the end of each segment, subsequently updating the position. Estimating power is essential; although the Cybertruck is rated at 845 hp, adjustments may be necessary.
Using 60% of the listed power, I found the Cybertruck’s eighth-mile time with the 911 in tow to be 8.31 seconds at 36.3 m/s. While this seems slow, it’s challenging to match both time and final speed with this basic model.
Applying the same model to the quarter-mile track, I generated a speed versus distance plot for the Cybertruck both with and without the 911.
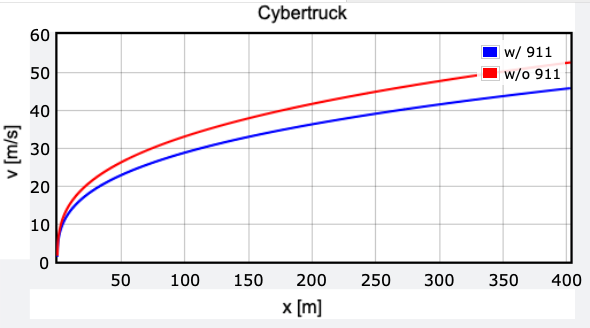
Based on this analysis, the Cybertruck without the 911 achieves a quarter-mile time of 11.49 seconds (impressive), whereas with the towing 911, the time extends to 13.19 seconds. Comparing this to the 911’s time of 11.5 seconds suggests that the Cybertruck would indeed fall short.
Perhaps this is the reason the race was conducted over just an eighth-mile distance. Just a thought.
Chapter 2 Title
The Cybertruck vs. Porsche 911: A Closer Look
In this video, the discussion revolves around the dynamics of the Tesla Cybertruck compared to the Porsche 911 under various conditions, including towing scenarios.